Mastering The Art Of Solving Fractions: A Comprehensive Guide

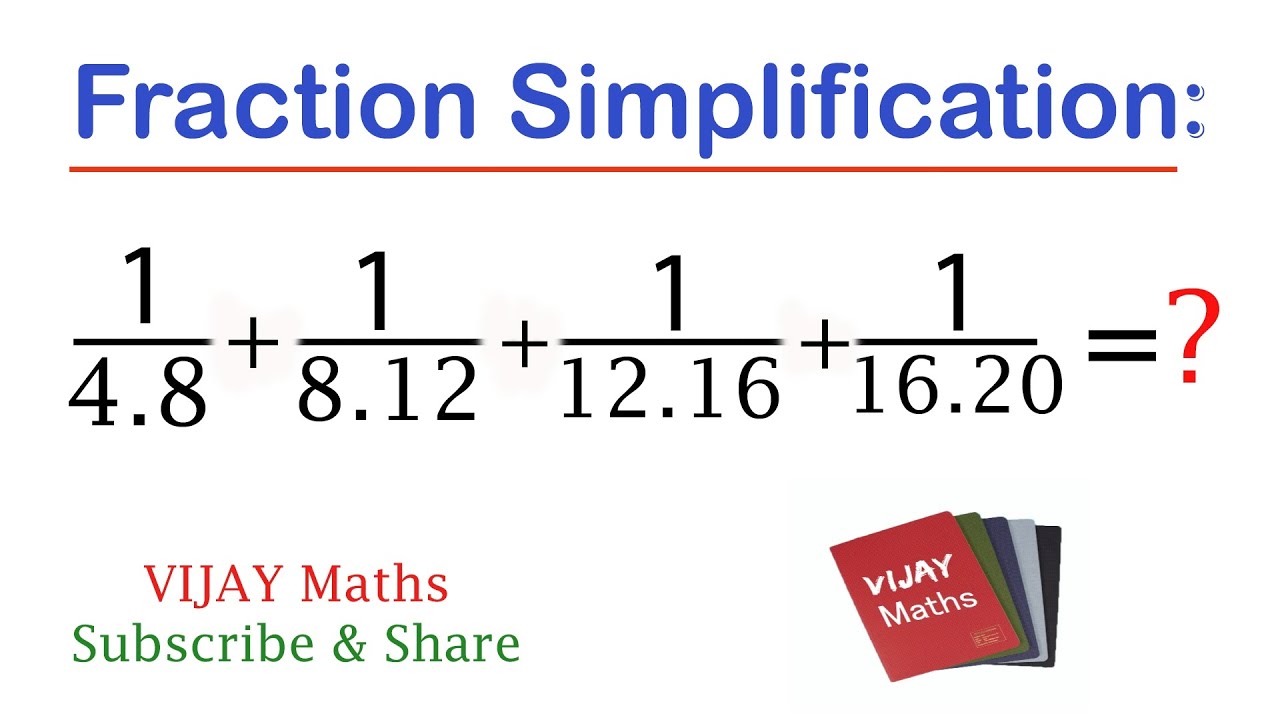
Understanding fractions can often feel like navigating through a complex maze of numbers and rules. However, with the right strategies and techniques, anyone can learn how to solve fraction problems effectively. Fractions represent parts of a whole, and mastering them is crucial in various fields, from cooking and carpentry to advanced mathematics and science. In this guide, we will explore the fundamental concepts of fractions, delve into different methods to solve them, and provide valuable tips to make the learning process easier.

Fractions consist of two main components: the numerator, which is the top number, and the denominator, the bottom number. The numerator indicates how many parts we have, while the denominator tells us how many equal parts the whole is divided into. This article aims to clarify these components and demonstrate how to solve fraction problems step by step, ensuring a solid understanding of the topic. Whether you are a student seeking help with your homework or an adult brushing up on your math skills, this guide is designed to cater to all levels.

As we embark on this journey to demystify fractions, we will tackle common questions such as “What are the different types of fractions?”, “How can I add or subtract fractions?”, “What about multiplying and dividing fractions?” and “Are there any tips for solving complex fraction problems?” By the end of this article, you will not only know how to solve fraction problems but also gain confidence in your mathematical abilities. Let’s dive in!

What Are the Different Types of Fractions?

Fractions can be classified into several categories based on their characteristics. Understanding these types is essential for effectively solving fraction problems. Here are the main types of fractions:

• Proper Fractions: These are fractions where the numerator is less than the denominator (e.g., 3/4).
• Improper Fractions: In these fractions, the numerator is greater than or equal to the denominator (e.g., 5/3).
• Mixed Numbers: These consist of a whole number and a proper fraction (e.g., 2 1/2).
• Equivalent Fractions: Different fractions that represent the same value (e.g., 1/2 and 2/4).

How to Solve Fraction Addition and Subtraction?

Adding and subtracting fractions might seem daunting at first, but it becomes manageable with practice. Here are the steps to follow:

Step 1: Finding a Common Denominator

To add or subtract fractions, you need to have a common denominator. If the denominators are the same, you can proceed to the next step. If not, find the least common denominator (LCD) by identifying the smallest multiple that both denominators share.

Step 2: Adjusting the Fractions

Once you have identified the common denominator, adjust the numerators accordingly. Multiply the numerator and denominator of each fraction by the necessary factor to ensure both fractions now have the same denominator.

Step 3: Adding or Subtracting the Numerators

With the fractions adjusted, you can simply add or subtract the numerators while keeping the common denominator. Finally, simplify the resulting fraction if possible.

Example of Fraction Addition

Let’s add the fractions 1/4 and 1/6:

• Find the common denominator (12).
• Adjust the fractions: (1/4 = 3/12 and 1/6 = 2/12).
• Add the numerators: 3 + 2 = 5, keeping the common denominator: 5/12.

What About Multiplying and Dividing Fractions?

Multiplication and division of fractions are generally more straightforward than addition and subtraction. Here’s how to approach each:

How to Multiply Fractions?

To multiply fractions, follow these steps:

• Multiply the numerators together to get the new numerator.
• Multiply the denominators to get the new denominator.
• Simplify the resulting fraction if possible.

Example of Fraction Multiplication

Multiply 2/3 by 3/4:

• Numerator: 2 × 3 = 6.
• Denominator: 3 × 4 = 12.
• Result: 6/12, which simplifies to 1/2.

How to Divide Fractions?

To divide fractions, you can use the following method:

• Flip the second fraction (take its reciprocal).
• Multiply the first fraction by this flipped fraction.
• Simplify the resulting fraction if possible.

Example of Fraction Division

Divide 2/3 by 3/4:

• Flip the second fraction: 4/3.
• Multiply: 2/3 × 4/3 = 8/9.

Are There Any Tips for Solving Complex Fraction Problems?

Complex fraction problems can be intimidating, but these tips can help you tackle them with confidence:

• Break down the problem into smaller, manageable parts.
• Always simplify fractions as you go to make calculations easier.
• Practice regularly to become more familiar with different types of fraction problems.
• Use visual aids, such as fraction bars or pie charts, to better understand the concepts.

How Can I Practice Solving Fractions?

Practice is essential to mastering fractions. Here are some effective ways to enhance your skills:

• Work on worksheets that focus on fractions, which can be found online or in math textbooks.
• Use educational apps or websites that offer interactive fraction games and quizzes.
• Join a study group where you can collaborate with others and learn from each other.
• Ask for help from a teacher or tutor if you are struggling with specific concepts.

Conclusion: How to Solve Fraction with Confidence

In conclusion, knowing how to solve fraction problems is a vital skill that can benefit you in many areas of life. By understanding the different types of fractions, mastering addition, subtraction, multiplication, and division techniques, and practicing regularly, you can build your confidence and improve your mathematical abilities. Remember to approach complex problems step by step and utilize the tips and resources available to you. With dedication and practice, you’ll become proficient in solving fractions and tackling more advanced mathematical challenges.